仿射的

These observations allow one to formalize the definition of reflection: a reflection is an involutive isometry of an Euclidean space whose set of fixed points is an affine subspace of codimension 1.

这些观察允许我们形式化反射的定义: 反射是欧几里得的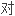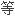距同构，它的不动点集是余维度为 1 的仿射子。